Do the math. Reject the WEF global resetters!

Bud Bromley | August 3, 2022

Do the math.

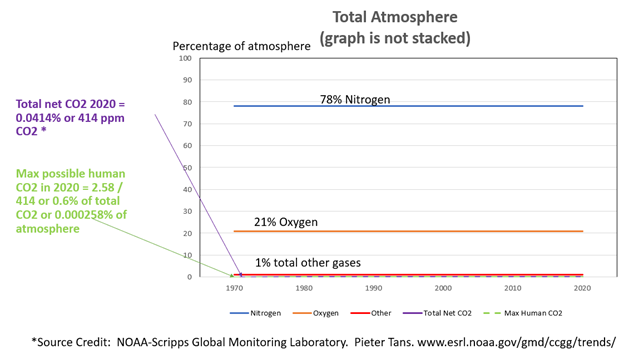
• Net CO₂ in air for 2020 was ~414 ppm, that’s 0.000414 *
• Human CO₂ cannot exceed 2.58 ppm, that’s 0.00000258, i.e., the net CO₂ increase due to all sources & sinks for year 2020 = 2.58 ppm. Net human CO₂ cannot exceed 0.000258% of atmosphere. *
• 2.58/414 = 0.0062 = 0.62% Net human CO₂ cannot exceed 0.62% of net total CO₂ for 2020.
• In 1970, average net CO₂ was 325.68 ppm *
• In 1969, average net CO₂ was 324.62 ppm *
• 325.68 minus 324.62 = 1.06 ppm *
• For 2020, average net CO₂ was 414.24 ppm *
• For 2019 average net CO₂ was 411.66 *
• 414.24 minus 411.66 = 2.58 ppm *
• 50 year maximum possible net human CO₂ increase = 2.58 minus 1.06 = 1.52 ppm
• Increase in 50 years total net CO₂ = 414.24 minus 325.68 = 88.56 ppm = 1.77 ppm/yr
• Slope for total net CO₂ = y = mx+b
• 414.24 = m50 + 325.68
• (414.24-325.68)/50 = m = 1.77 ppm/year average
• Slope for 50 year maximum possible human CO₂ increase y = mx + b
• (2.58 – 1.06)/50 = m = 0.0304 ppm/yr average
• 1.77 / 0.0304 = 58
• Total net CO₂ growing 58 times faster than maximum possible human CO₂.
• Therefore, human CO₂ is not causing growth in global CO₂.

It is maximum possible net human CO₂ because the annual increase from 1969 to 1970 (i.e., 1.06 ppm), and the annual increase from 2019 to 2020 (i.e., 2.58 ppm), includes the increase in CO₂ due to all CO₂ sinks and all CO₂ sources, human and natural, for those two years. There is no CO₂ “atmospheric fraction” hidden or accumulating somewhere.

As stated previously on this blog, if net human CO₂ emissions were causing the slope in net global average atmospheric CO₂ concentration, then the two slopes must be parallel or intersect, that is, there must be a positive correlation. However, the two slopes are diverging over time, the correlation is negative, therefore net human emissions cannot be the cause of the increasing slope of net global average atmospheric concentration.

Using these same data 1970-2020, annual averages, there is an apparent increase of about 88.56 ppm (414.24 – 325.68) over the 50 years, which is only about 1.77 ppm per year.  This is already a small amount and probably would be lost in the noise of the two giant fluxes, i.e., c absorption flux and CO₂ emission flux.  But even that 1.77 ppm/yr needs further investigation.  For example, there is the larger seasonality (i.e., the sharks teeth seasonal cycle) which has larger variation at some of the sampling locations than the 1.77 ppm/yr, but not at other locations.  And no doubt there are other cycles to be found in the data, el Nino’s for example, and human population differences driving CO₂ emission differences between northern and southern hemispheres.

Is this 1.77 ppm/yr a net accumulation of CO₂?  Or is this an adjustment of the partition ratio resulting from the phase-state reaction and partition of CO₂ gas between air vs the environment, mostly ocean surface?  Or, is the phase-state partition ratio perturbed by a trend in sea surface temperature? Or, perhaps perturbed by temperature, salinity, alkalinity or partial pressure changes at the measurement location?

NOAA-Scripps averages averages of averages, as they acknowledge in the footnote of the table in one of their papers copied below.  It is well known that this is bad practice and results in errors.  But it depends on how exactly it is done.  To figure this out is time consuming, as you can imagine.

For example: the average of {2,2,2,2,2,2,2,2,2,2,2,2,2} is 2, (N=13) and the average of {4} is 4. (N=1). The average of the averages is 3. But the average of all numbers is 30/14 ≃ 2.14.  It is possible to produce a trend in the data by averaging averages post data collection.

It is curious that they report – for 60 years – uncertainty of each annual mean of exactly 0.12. They define uncertainty, “The estimated uncertainty in the annual mean is the standard deviation of the differences of annual mean values determined independently by NOAA/ESRL and the Scripps Institution of Oceanography.”

Is this apparent trend in CO₂ concentration of 1.77 ppm per year real?  And, what are the effects of suppression of the variance in the data due to the averaging of averages?

References:

Thoning, K., Tans, P., Komhr, W. 1989.  Atmospheric carbon dioxide at Mauna Loa Observatory: 2. Analysis of the NOAA GMCC data, 1974-1985.  Journal of Geophysical Research.

Abstract: The first 12 years (1974–1985) of continuous atmospheric CO/sub 2/ measurements from the NOAA GMCC programs at the Mauna Loa Observatory in Hawaii are analyzed. Hourly and daily variations in the concentration of CO/sub 2/ due to local sources and sinks are described, with subsequent selection of data representing background concentrations. A digital filtering technique using the fast Fourier transform and low-pass filters was used to smooth the selected data and to separate the seasonal cycle.

Tans, P. CO₂ annual means 1959 -2020. NOAA and Scripps. Global Monitoring Laboratory. File Creation: Fri Mar 5 08:40:06 2021. http://www.esrl.noaa.gov/gmd/ccgg/trends/